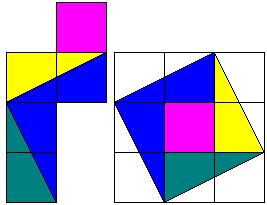
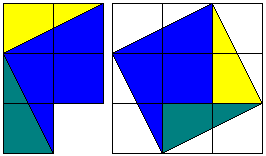
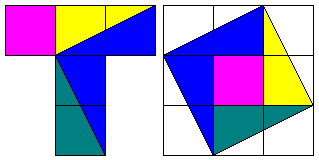
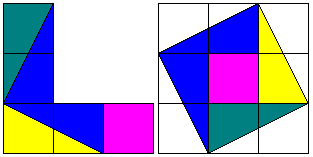
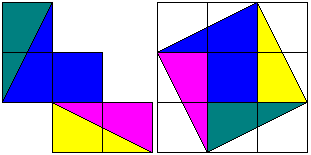
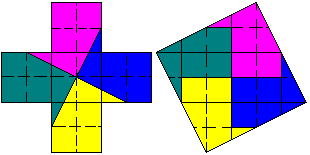
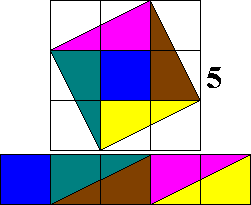Since the surface of one pentomino is 5, we must make a square with area 5.
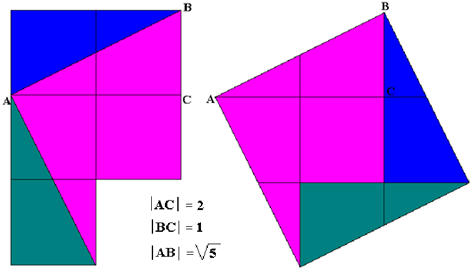
We can get 5 as the sum of the squares of 1 and 2. So if we have a right triangle with sides 1 and 2 then the hypotenuse is the side of a square with area 5.
OThe drawing below is from our syllabus of NWD 2002
 |
 |
|
|
|
|

|
|
|

|
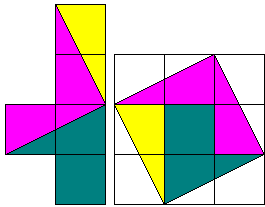 |
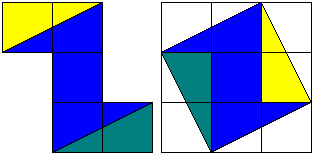 |
Aad van de Wetering mailed us in August 2000 :"Hoewel ik de oplossing in 5 stukjes veel en veel mooier vind, is er een lelijke oplossing met slechts 4 stukjes mogelijk. De oplossing in vijf kun je voor alle pentomino's (behalve X) gebruiken en 't is zo mooi symmetrisch"
|
|
|
On the
site of Giovanni Resta are dissections from pentominoes
to tetrominoes.
There is a dissection of Livio Zucca of the X-pento to a square in four pieces.
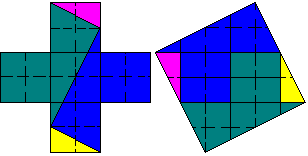
From
Helmut Postl (Wenen) we got a beautiful dissection of the X-pento also in four
pieces
Thanks
Comments mail to
o.d.m@fulladsl.be
We have made a geogebra animation of a
Y-pentomino-dissection.
In Battambang (Cambodia) the monks can made of the P-pentomino
a square.
Roel Huisman made us 3 animations
P-pento
Y-pento
Z-pento
You can find it also on http://www.fi.uu.nl/nwd by handouts 2004
The best example is cutting a square cake to a X-pentomino, a problem of Peter Hendriks.