Pieter Torbijn asked to Aad van de Wetering to mail us this nice pentominoprobleem.
We asked Dario permission to put it on the site and his answer was: "Yes, Ciao, Dario"
Place four of the twelve pentominoes in the shape so that it is no longer possible to put one of the remaining 8 pentominoes in this shape.
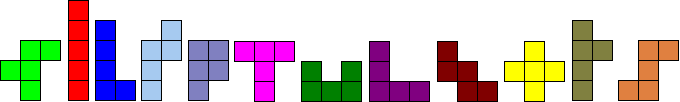

Pieter Torbijn saw the solution immediately. We need about an hour to find the solution as well as Aad van de Wetering.
Would you like to know which four you should use?
Helmut Postl send us a solution: "The problem from Dario is really a nice one. At the first moment it seems impossible to solve, but the trick is to leave spaces just in the shape of the already used pentominoes. I think the solution is unique, but I don't know for sure."
We also believe that there is only one solution and Dario confirms it:"I think that only this solution exist."
George Sicherman: "This problem does indeed have a unique solution, allowing reflection. Dario and Helmut express uncertainty about this. I have checked all possible placements of the pentominoes, and there is only one solution"
Thanks to George to check it.