|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
2 |
N | Y | Y | Y | Y | Y | N | Y | N | N | N | N |
|
3 |
Y | N | N | N | Y | Y | N | N | N | Y | Y | N |
|
4 |
Y | N | N | Y | Y | N | N | Y | Y | N | N | Y |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
2 |
Y | N | N | Y | N | Y | Y | N | Y | N | Y | Y |
|
3 |
N | N | Y | N | N | Y | N | N | N | Y | Y | Y |
|
4 |
N | N | N | Y | N | N | Y | Y | N | N | N | Y |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | Y | Y | Y | Y | N | Y | N | N | N | N |
|
2 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
3 |
N | Y | Y | Y | N | N | Y | Y | Y | N | N | Y |
|
4 |
Y | N | Y | N | Y | N | N | Y | Y | N | N | N |
|
|
|
Each scheme uses the relative directions of the pentomino ends
in 2 of the 4 classification criteria, so I should explain that these "ends" and
their "directions" are the same as in Martin Watson's recent Pentomino Pipes
challenge.
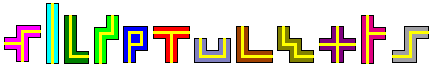
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | Y |
|
2 |
Y | N | N | Y | Y | Y | Y | Y | N | N | N | Y |
|
3 |
Y | N | Y | N | N | Y | N | Y | Y | Y | Y | N |
|
4 |
Y | Y | N | N | Y | Y | N | N | N | Y | Y | Y |
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
3 |
Y | N | Y | N | N | Y | N | Y | Y | Y | Y | N |
|
4 |
Y | Y | N | N | Y | Y | N | N | N | Y | Y | Y |
|
5 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
6 |
N | N | Y | N | N | Y | Y | Y | N | N | Y | Y |
Bob Henderson mails us:"I found other classifications that
depend on the letter of the alphabet each pentomino resembles, but I think that
the geometric categories are less arbitrary."
He had the same idea as Martin Watson.
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
2 |
N | N | N | Y | Y | Y | Y | Y | N | N | N | N |
|
3 |
N | Y | Y | Y | Y | N | Y | Y | Y | N | N | Y |
|
4 |
N | Y | Y | N | Y | N | Y | N | N | N | Y | N |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | Y | Y | Y | Y | N | Y | N | N | N | N |
|
2 |
Y | N | Y | N | Y | Y | Y | Y | N | N | Y | Y |
|
3 |
N | N | Y | Y | Y | N | Y | N | Y | N | N | Y |
|
4 |
Y | N | N | Y | Y | N | Y | Y | N | Y | N | N |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
Y | N | Y | N | Y | Y | Y | Y | N | N | Y | Y |
|
2 |
N | Y | Y | Y | Y | N | Y | N | N | N | Y | N |
|
3 |
Y | N | N | N | Y | Y | N | N | N | Y | Y | N |
|
4 |
N | N | N | Y | N | Y | Y | N | N | N | Y | Y |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
2 |
Y | N | N | N | Y | Y | Y | Y | Y | N | N | Y |
|
3 |
N | Y | N | Y | N | Y | Y | N | N | N | Y | Y |
|
4 |
N | N | N | Y | Y | N | Y | N | Y | N | N | Y |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
2 |
N | Y | Y | Y | N | N | Y | Y | Y | N | N | Y |
|
3 |
N | Y | Y | Y | Y | N | Y | N | N | N | Y | N |
|
4 |
Y | N | N | Y | Y | N | Y | Y | N | Y | N | N |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | Y | N | N | N | Y | Y | Y | Y | Y | N | N |
|
2 |
Y | N | N | N | N | Y | N | Y | Y | Y | N | Y |
|
3 |
N | Y | Y | Y | N | N | Y | Y | Y | N | N | Y |
|
4 |
N | Y | Y | N | Y | Y | N | Y | N | N | N | N |
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
| N | Y | Y | Y | N | N | Y | Y | Y | N | N | Y | |
| N | N | Y | N | Y | Y | Y | Y | N | N | Y | N | |
| N | Y | Y | N | N | Y | N | Y | N | Y | Y | Y | |
| N | Y | N | N | N | Y | Y | Y | Y | Y | N | N | |
| N | Y | Y | Y | Y | Y | N | Y | N | N | N | N | |
| N | Y | Y | Y | Y | N | Y | N | N | N | Y | N | |
| Y | N | N | N | Y | Y | Y | Y | Y | Y | N | Y | |
| N | N | Y | N | Y | Y | Y | Y | Y | N | Y | N | |
| Y | N | N | N | N | Y | N | Y | Y | Y | N | Y | |
| N | Y | Y | N | N | N | Y | N | N | N | N | Y | |
| Y | N | N | N | Y | Y | Y | Y | Y | N | N | Y | |
| N | N | Y | Y | Y | N | Y | Y | Y | N | Y | N | |
| N | Y | Y | Y | Y | N | N | N | N | N | Y | N | |
| Y | N | Y | Y | N | Y | Y | N | N | N | Y | Y | |
| Y | N | Y | Y | N | Y | N | N | N | N | Y | Y |
With these 15 questions there are 1365 different possible combinations of 4 questions, of which only 29 different combinations each uniquely determine the pentominoes:
J. Viljoen
mails us:"Herewith my lazy solution.Yes, I know it is cheating. Or is it? It
seems to meet the specifications of the challenge, at least the way I read them."
Alphabetise the pentominoes as is the custom: F I L N P T U V W X Y Z. Now,
using this alphabetical order, number them from 1 to 12. F will therefore be 1,
and Z will be 12. Turn this number into a 4-bit binary number.
|
|
|
|
|
F :0001 | I:0010 | L:0011 | N:0100 | P:0101 | T:0110 | U:0111 | V:1000 | W:1001 | X:1010 | Y:1011 | Z:1100 |
|
1 |
Y | N | Y | N | Y | N | Y | N | Y | N | Y | N |
|
2 |
N | Y | Y | N | N | Y | Y | N | N | Y | Y | N |
|
3 |
N | N | N | Y | Y | Y | Y | N | N | N | N | Y |
|
4 |
N | N | N | N | N | N | N | Y | Y | Y | Y | Y |
|
|
|
|
|
F | I | L | N | P | T | U | V | W | X | Y | Z |
|
1 |
N | N | N | N | N | N | N | Y | Y | Y | Y | Y |
|
2 |
N | N | N | Y | Y | Y | Y | N | N | N | N | Y |
|
3 |
N | Y | Y | N | N | Y | Y | N | N | Y | Y | N |
|
4 |
Y | N | Y | N | Y | N | Y | N | Y | N | Y | N |
Martin mails us: "This probably isn’t the kind of answer you
wanted, but I like it. I am sure your class will enjoy it!!
This is effectively allocating each pentomino a binary number from F=0001 upto
Z=1100. Each ‘1’ corresponds with a YES.
Every pentomino has a different answer. This would still work if there were 16
pentominoes, from 0000 to 1111."
We have nothing to add to this.














