With many thanks to Pieter Torbijn for this problem.

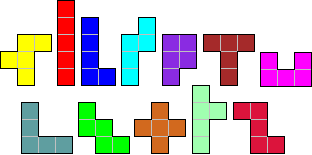
Start with a
set of pentominoes
Symmetry from 1 up to 12 and back
|
With many thanks to Pieter Torbijn for this problem. |
Start with a
set of pentominoes |
Assign numbers to the 12
pentominoes from 1 up to 12
Make 2 series of 12 shapes, all containing at least one axis of symmetry,
with the following conditions:
The shapes may not contain any holes.
Series 1: pentomino 1; pentominoes 1+2; ... ;pentominoes 1+2+...+12.
Series 2: pentomino 12; pentominoes 12+11; ... ;pentominoes 12+...+2+1.
This will be made clearer in the following example.
This example is not a correct solution, as holes are not allowed!
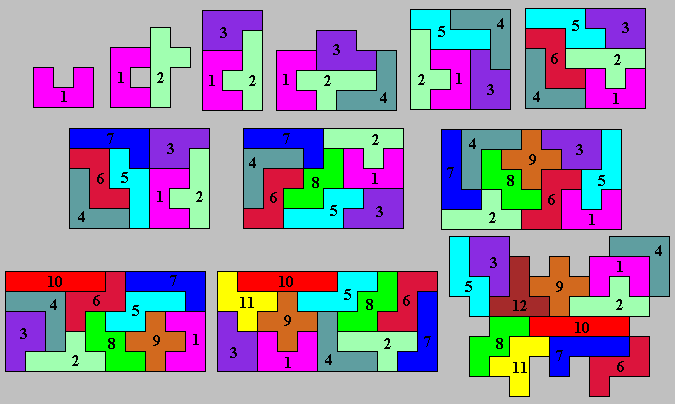
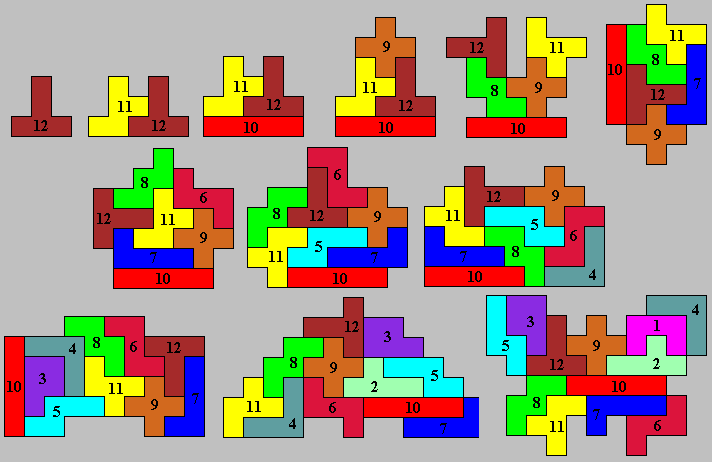
Reinhard Grafl
from Austria sent us the last shape, a butterfly.
We started with the U-pentomino and ended with the T-pentomino. The more
correct examples you send, the larger the chance that you’ll become the
winner.
In case you want an extra challenge, then we invite you to look for
solutions for which the total perimeter of all 23 different shapes is either
maximal or minimal. (The shape containing 12 pentominoes is counted only
once now).
For solving the problem Exel can be used. We have a
file
containing the pentominoes.
![]()