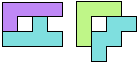Symmetrical enclosure

Aad Thoen sent me an e-mail with the following challenge:
Make a as large as possible symmetrical (inside as well as outside) enclosure with a diagonal axis of symmetry.
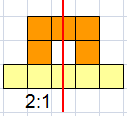
Here an example with 7 pentominoes and enclosure of 31.

However we extend the challenge to enclosures with any axis of symmetry (diagonal or orthogonal).

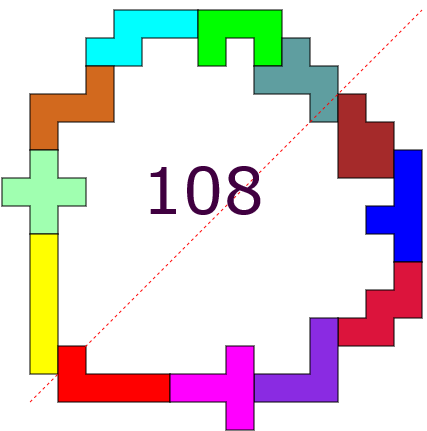
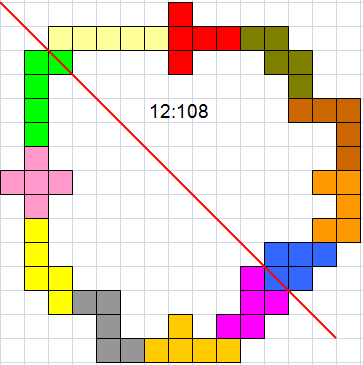
Aad has enclosed 85 units with 11 pentominoes. Colors have been added to the figure to make the diagonal symmetry more visible.
Aad found also solutions with enclosures of 48, 51 and 54. The breakthrough to  85 came when he discovered how to let T and X correspond.
 
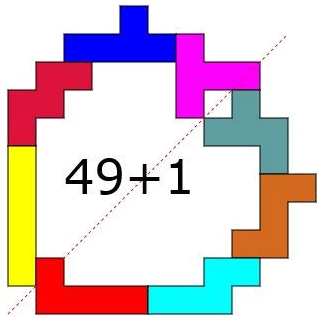
With 2 pentominoes we have enclosed 1 unit.


(HP)


(HP)

All possible figures consisting of 2 different pentominoes with an axis of symmetry are contained in another page of this site.

 With 3 pentominoes .

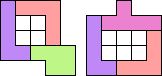
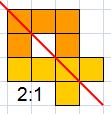
George Sicherman:"I think I set the record for four pentominoes with a diagonally symmetric!"

 11 units are enclosed.

 We also admit orthogonal symmetry, as long as any axis of symmetry is present!


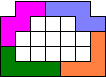
 With 5 pentominoes


23 (OM and HP)


21 (GS and HP)

With 6 pentominoes


Aad Van de Wetering
(and HP)


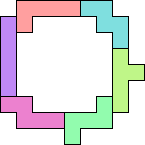
ILNWYZ
32(GS)


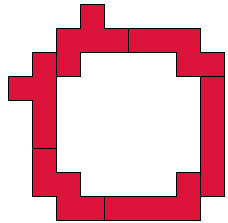
FILNWY
32(AT)

With 7 pentominoes


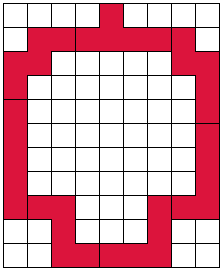
46 (AT)


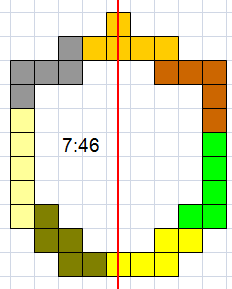
Helmut Postl


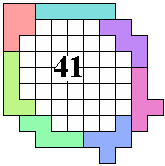
George Sicherman


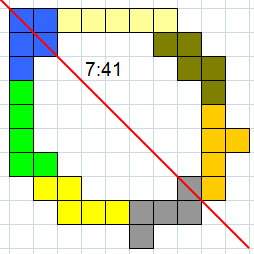
Helmut Postl

With 8 pentominoes


Aad van de Wetering


Helmut Postl


54(OM)


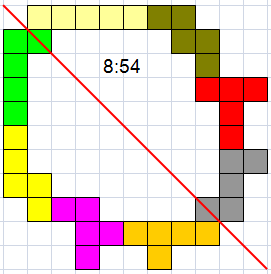
Helmut Postl

With 9 pentominoes


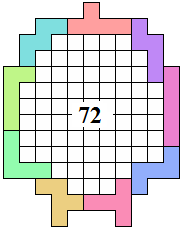
George Sicherman


Helmut Postl


George Sicherman


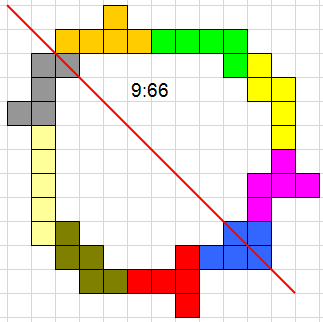
Helmut Postl

With 10 pentominoes
Aad Thoen: "Thanks to the FT combination of GS I join the game again."  This is why the solution of George is still present.


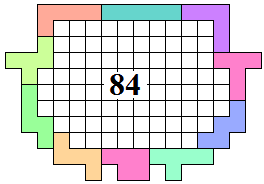
George Sicherman


Aad Thoen

But here is Helmut!

With 11 pentominoes


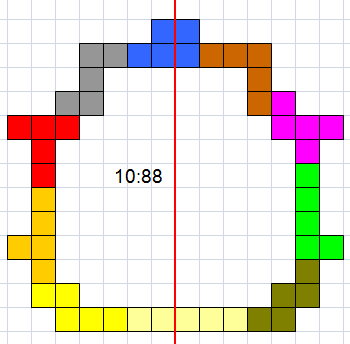
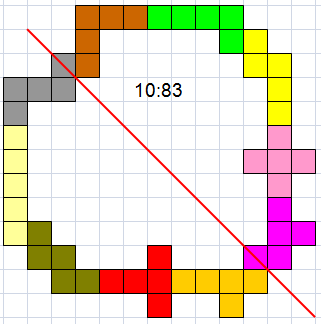
(OM, AW and HP)


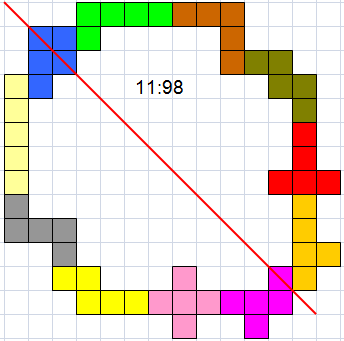
Helmut Postl

With 12 pentominoes
 The 3 latest results follow:


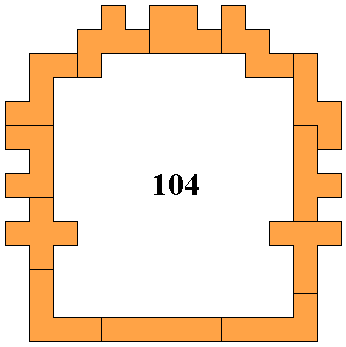
Aad Thoen


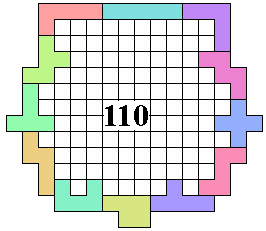
George Sicherman


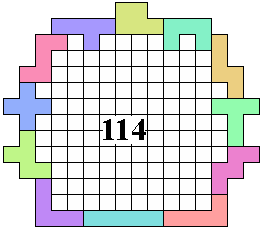
Odette De Meulemeester
 (found 2016)

From Rodolfo Kurchan we got a picture of the front cover of Puzzle Fun of April 1995.

 This record apparently is on the credit of Mike Reid.
L'histoire se répète (for insiders and our kubusman ;-)
Our solution is not completely identical and we are thrilled with the finding.
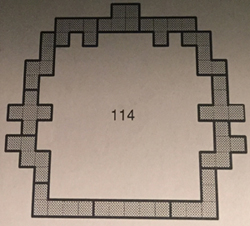
Helmut Postl also found 114 but in a different way:

 

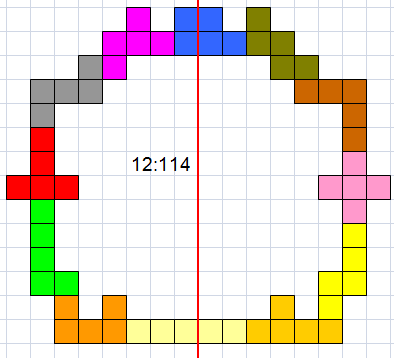
 Aad van de Wetering


Helmut Postl


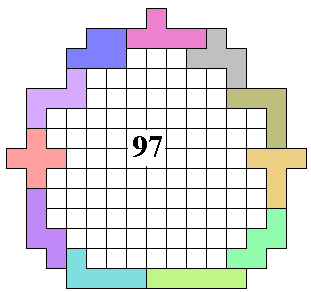
With this set Aad van de Wetering searches for all his records

 We are looking for such a magical set ;-)

Hieronder nog enkele omsluitingen die uit verschillende delen bestaan.


+1 is discutabel
 Dat extra vakje ligt zeker en vast binnen de buitenomtrek. Ook buiten de binnenomtrek, maar wie maalt daarom....
 38+1=38(AW)  


(AW)


(OM)

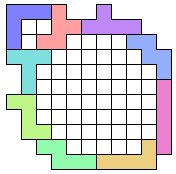
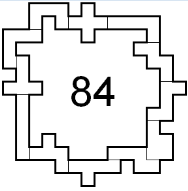
Edo Timmermans had already earlier (on the occasion of contest 51) sent an enclosure symmetrical on the in- and outside with all pentominoes.

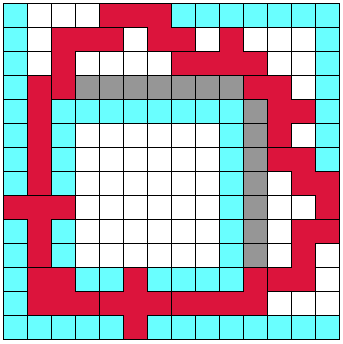
 He also sent fencings with 8 pentominoes which are the enfolding of an empty box.
Edo further searched for fencings with the hexominoes which are the enfolding of a cube.


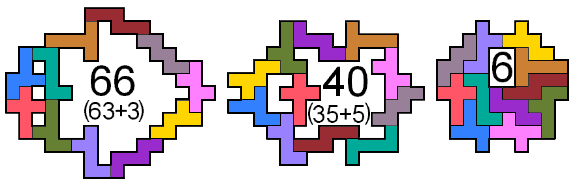
 Did you find any other enclosures with an axis of symmetry?