F, P , I and L

The U can be in 2 positions

 

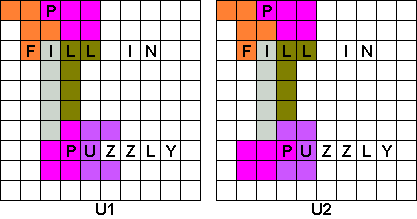
We continue with U1.
Z can in 2 positions

 

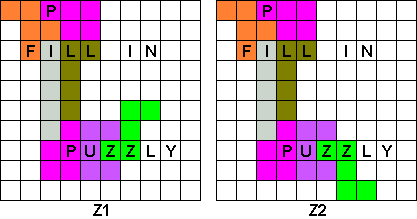
 Z1.

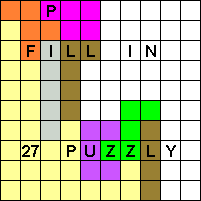
Either way, the L-pento closes an area of ​​27 squares. This is not a multiple of 5.
Z1 is therefore not possible.

 Z2.

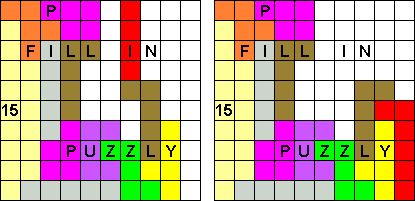
This is not possible because of the red part.
U2 is the correct position.

 P to the left. Then only I, L and V.

Z1 is not possible (red square)
We continue with Z2

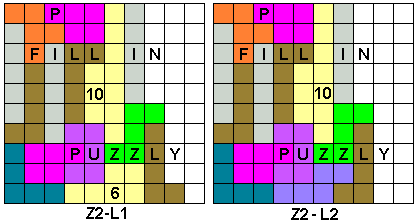
L1 is not possible because L1 closes area of ​​6 squares.
L2 is not possible because we cannot place Y anymore.
So it must be Z3.

Y is not possible with L1.

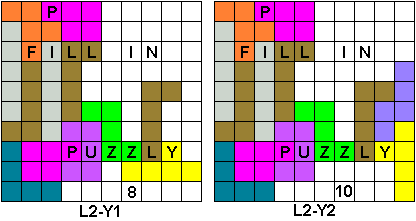
We can place Y by L2.
Y1 closes area of ​​8 squares. Y1 is wrong.
Y2 closes area of ​​10 squares that can be filled with NP or with YP.
We continue with Y2

I1 and I2 are not possible, because of the red area.
At I3 we can no longer place the N.
L2 is therefore not possible, so it must be L3.

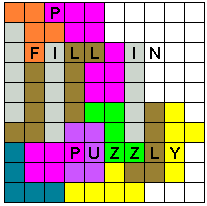
We put the Y

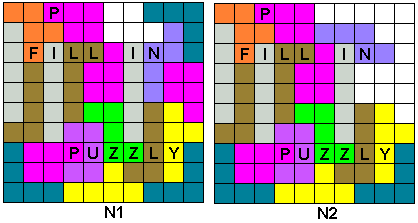
By N1:  P lie against each other.
So it must be N2.
We continue with I, U and Z.